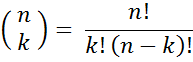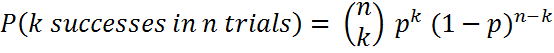Ans: Compute the weighted average of the outcomes, weighted by the probability of each outcome:
E(x) = x1 p1 + ... + xn pn
Ans: The long run average of the outcomes of the random variable over a very large number of trys.
E(x) = x1 p1 + x2 p2 = 0(1 - p) + 1p = p
Ans: If x is a Bernoulli random variable,
Var(x) = (x1 - E(x))2 P(x1) + ... + (xk - E(x))2 P(xk)
= (0 - p)2 (1 - p) + (1 - p)2 p = p(1 - p)(p + 1 - p) = p(1 - p)
Then
σx = √Var(x) = √p(1 - p)
- getting at exactly 5 heads in a row?
Ans: (1/2)6 = 1/64. The sequence must be HHHHHT - getting at least 5 heads in a row?
Ans: (1/2)5 = 1/32. The sequence must be HHHHH; we don't care what the next outcome is. - obtaining the sequence THTTH?
Ans: (1/2)5 = 1/32
- Roll a die 4 times. What is the probability of getting at least one
ace (1)?
Ans: 1 - (1 - 1/6)4 = 0.5177 = 51.8% - Roll a pair of dice 24 times.
What is the probability of obtaining snakeeyes (pair of aces
at least once)?
Ans: 1 - (1 - 1 / 36)24 = 0.4914 = 49.1%
Ans: E(S) = nE(x) = 100(15) = 1,500.
Ans: σS = √n σx = √100 3 = 30.
If you bet on red or black, here are the payoff tables for an American roulette wheel:
| Bet on Red | ||
|---|---|---|
| Outcome | Payoff | Probability |
| Red | +1 | 18/38 = 0.474 |
| Black | -1 | 18/38 = 0.474 |
| Green | -1 | 2/38 = 0.052 |
| Bet on Black | ||
|---|---|---|
| Outcome | Payoff | Probability |
| Red | -1 | 18/38 = 0.474 |
| Black | +1 | 18/38 = 0.474 |
| Green | -1 | 2/38 = 0.052 |
What is the expected payoff of playing on red? playing on black.
Ans: Playing on red is E(x) = 1(0.474) + (-1)(0.474) + (-1)(0.052) = -0.052. You lose about 5 cents each time you play on red, same for black.
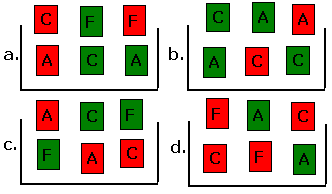
Ans: In a and b, letter is independent of number. To show that letter
and number are independent, you need to show that for each letter value L
P(L) if you don't know the color = P(L) for a given color.
For example, in Problem 8a, if you don't know the color,
P(A) = P(B) = P(C) = 2/6 = 1/3.
If you know the color is red, P(A) = P(B) = P(C) = 1/3. If you know the
color is green, the probabilities are also 1/3.
In Problem 8c, if you don't know the color, P(F) = 2/6 = 1/3. If you know that the color is green, P(F) = 2/3 = 2/3, so color and letter are not independent.
To show that two events are not independent, it is enough to find one case where the probabilities are different; to show that two events are independent, you must show that the probabilities are the same for all choices of color and letter.
Person A lives at least 20 more years after February 21, 2011.
Person B lives at least 20 more years after February 21, 2011.
Ans: It is hard to know. If the people know each other, their lives and life choices can affect each other so A and B are probably not independent. If the people do not know each other, A and B may be independent, but they may also be related in unexpected ways.
Ans: (1 /300,000)2 = 1 / 90,000,000,000.
Ans: 1 - (1 - p)^n = 1 - (1 - 0.03)^52 = 0.7948 = 79%.
Ans: If A and B are independent, P(A and B) = P(A) P(B). But if A and B are mutually exclusive, P(A and B) = 0. Thus P(A) P(B) = 0 and either P(A) = 0 or P(B) = 0.
Ans: First estimate the probability of a hole in one in a single try. The area of the hole in square feet ipi*r2/144 = pi*4.252/144 =0.3757 ft2. If the area of the green is 4000 ft2, the probability of a hole in one is 0.1 * 0.3757 / 4000 = 9.3925 × 10-6. This the probability of getting at least one hole in one in 50 years is
1 - (1 - 9.3925 × 10-6)(50 * 52) = 0.024 = 2.4%